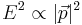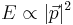Superfluid vacuum

Beyond the Standard Model
Standard Model
Quantum gravity
String theory
Loop quantum gravity
Causal dynamical triangulation
Canonical quantum gravity
Superfluid vacuum theory

Superfluid vacuum theory (SVT), sometimes dubbed as the theory of BEC vacuum, is an approach in theoretical physics and quantum mechanics where the physical vacuum (fundamental non-removable background) is viewed as a superfluid or BEC. The microscopical structure of the vacuum is currently largely unknown and is a subject of intensive studies in SVT. The ultimate goal of the approach is to develop scientific models that unify quantum mechanics (describing three of the four known fundamental interactions) with gravity. This makes SVT a candidate for the theory of quantum gravity. It is hoped that development of such theory would unify into a single consistent model all fundamental interactions, and to describe all known interactions in the Universe, at both microscopic and astronomic scales, as different manifestations of the same entity, superfluid vacuum.

Contents

History

The concept of a luminiferous aether as a medium sustaining electromagnetic waves was discarded after the advent of the special theory of relativity. The aether, as conceived in classical physics, does indeed lead to several contradictions; in particular, aether having a definite velocity at each space-time point will exhibit a preferred direction. This is in conflict with the relativistic requirement that all directions within the light cone are equivalent. However, as early as in 1951 P.A.M. Dirac published two papers[1][2] where he pointed out that we should take into account quantum fluctuations in the flow of the aether. His arguments involve the application of the uncertainty principle to the velocity of aether at any space-time point, implying that the velocity will not be a well-defined quantity. In fact, it will be distributed over various possible values. At best, one could represent the aether by a wave function representing the perfect vacuum state for which all aether velocities are equally probable. Although these works didn't gain much popularity, they can be regarded as the birth point of the theory.

Inspired by the Dirac ideas, K.P. Sinha, C. Sivaram and E.C.G. Sudarshan published in 1975 a series of papers that suggested a new model for the aether according to which it is a superfluid state of fermion and antifermion pairs, describable by a macroscopic wave function.[3][4][5] They correctly noticed that particle-like small fluctuations of superfluid background do obey the Lorentz symmetry even if the superfluid itself is non-relativistic. Nevertheless, they decided to treat the superfluid as the relativistic matter - by putting it into the stress-energy tensor of the Einstein equations, etcetera. This did not allow them to make an important step - describe the relativistic gravity as one of the small fluctuations of the superfluid vacuum as well. This was done by other authors subsequently.

Since then, several theories have been proposed within the SVT framework. They share the main idea but differ in how the structure and properties of the background superfluid must look. In absence of observational data which would rule out some of them, these theories are being pursued independently.

Relation to other concepts and theories

Lorentz and Galilean symmetries

According to the approach, the background superfluid is assumed to be essentially non-relativistic whereas the Lorentz symmetry is not an exact symmetry of Nature but rather the approximate description valid only for small fluctuations. An observer who resides inside such vacuum and is capable of creating or measuring the small fluctuations would observe them as relativistic objects - unless their energy and momentum are sufficiently high to make the Lorentz-breaking corrections detectable.[6] If the energies and momenta are below the excitation threshold then the superfluid background behaves like the ideal fluid, therefore, the Michelson-Morley-type experiments would observe no drag force from such aether. Also, the Lorentz-symmetric models are obviously a good approximation in that case. However, in the close vicinity of the threshold the relativistic description begins to fail: of course, as one approaches higher and higher energy scales one can still employ the relativistic description as an effective one but the price will be that it will become more and more "effective" and less and less natural since one will need to adjust the form of the covariant field-theoretical action by hand.

Further, in the theory of relativity the Galilean symmetry (pertinent to our macroscopic non-relativistic world) arises as the approximate one - when particles' velocities are small compared to speed of light in vacuum. In SVT one does not need to go through Lorentz symmetry to obtain the Galilean one - the dispersion relations of most non-relativistic superfluids are known to obey the non-relativistic behavior at large momenta.[7][8][9]

To summarize, vacuum superfluid describes relativistic objects at "small"[nb 1] momenta (a.k.a. the "phononic" limit)

E^2 \propto |\vec p|^2

and non-relativistic ones

E \propto |\vec p|^2

at large momenta. The most interesting nontrivial physics is believed to be located somewhere between these two regimes.

Relativistic quantum field theory

In the relativistic quantum field theory the physical vacuum is also assumed to be some sort of non-trivial medium to which one can associate certain energy. This is because the concept of absolutely empty space (or "mathematical vacuum") contradicts to the postulates of quantum mechanics. According to QFT, even in absence of real particles the background is always filled by pairs of creating and annihilating virtual particles. However, a direct attempt to describe such medium leads to the so-called ultraviolet divergences. In some QFT models, such as quantum electrodynamics, these problems can be "solved" using the renormalization technique, namely, replacing the diverging physical values by their experimentally measured values. In other theories, such as the quantum general relativity, this trick does not work, and reliable perturbation theory cannot be constructed.

According to SVT, this is because in the high-energy ("ultraviolet") regime the Lorentz symmetry begins to fail, as mentioned above, so theories based on it cannot be regarded as valid for all scales of energies and momenta.

Curved space-time

According to general relativity, the gravitational interaction is described in terms of space-time curvature using the mathematical formalism of the Riemannian geometry. This was supported by numerous experiments and observations in the regime of low energies. However, the attempts to quantize general relativity led to various severe problems, therefore, the microscopical structure of gravity is still ill-defined. There may be a fundamental reason for it - the degrees of freedom the general relativity is based on may not be the correct ones but only approximate and effective. The question whether the general relativity is an effective theory has been raised long time ago.[10]

According to SVT, the curved space-time arises as the small-amplitude collective excitation mode of the non-relativistic background condensate.[6][11] The mathematical description of this is similar to fluid-gravity analogy which is being used also in the analog gravity models.[12] Thus, relativistic gravity is essentially a long-wavelength theory of the collective modes whose amplitude is small compared to the background one. Outside this requirement the curved-space description of gravity in terms of the Riemannian geometry becomes incomplete or ill-defined.

Cosmological constant

The notion of the cosmological constant makes sense in a relativistic theory only, therefore, within the SVT framework this constant can refer at most to the energy of small fluctuations of the vacuum above a background value but not to the energy of vacuum itself.[13] Thus, in SVT this constant does not have any fundamental physical meaning and the related problems, such as the vacuum catastrophe, simply do not occur in first place.

Gravitational waves and gravitons

According to general relativity, the conventional gravitational wave is:

  1. the small fluctuation of curved spacetime which
  2. has been separated from its source and propagates independently.

Theory of superfluid vacuum brings into question that the relativistic object possessing both of these properties may exist in Nature.[11] Indeed, according to the approach, the curved spacetime itself is the small collective excitation of the superfluid background, therefore, the property (1) means that the graviton would be in fact the "small fluctuation of the small fluctuation" which does not look like a physically robust concept (as if somebody tried to introduce small fluctuations inside a phonon, for instance). As a result, it may be not just a coincidence that in general relativity the gravitational field alone has no well-defined stress-energy tensor, only the pseudotensor one.[14] Therefore, the property (2) cannot be completely justified in a theory with exact Lorentz symmetry which the general relativity is. Though, SVT does not a priori forbid an existence of the non-localized wave-like excitations of the superfluid background which might be responsible for the astrophysical phenomena which are currently being attributed to gravitational waves, such as the Hulse-Taylor binary. However, such excitations cannot be correctly described within the framework of a fully relativistic theory.

Mass generation and Higgs boson

While SVT does not explicitly forbid the existence of the electroweak Higgs particle, it has its own idea of the mass generation mechanism - elementary particles acquire mass due to the interaction with the vacuum condensate, similarly to the gap generation mechanism in superconductors.[11][15] Although this idea is not entirely new, one could recall the relativistic Coleman-Weinberg approach,[16] SVT gives the meaning to the symmetry-breaking relativistic scalar field as describing small fluctuations of background superfluid rather than an elementary particle. This may result in inability to detect the Higgs boson as an elementary particle of the electroweak-scale mass. Also, some versions of SVT favor a wave equation based on the logarithmic potential rather than on the quartic one. The former potential has not only the Mexican-hat shape, necessary for the spontaneous symmetry breaking, but also some other features which make it more suitable for the vacuum's description.

String theory and supersymmetry

Superstring theory was originally designed as a set of the Lorentz-covariant quantum gravity models whose renormalizability was ensured by assuming superconformal symmetry and higher-dimensionality as fundamental properties of space-time. The theory postulates that some extended objects are more fundamental than point particles. String theory is known to suffer from various problems, not to mention that its non-perturbative formulation is still pending.

According to SVT, theory of quantum gravity, as many other physical theories we know, does not have to be renormalizable nor valid at all scales. Instead, SVT models have the ultraviolet cutoff scale which is determined by the vacuum energy and corresponding length scale - such that all practical computations will necessarily contain this finite cutoff. Besides, as long as the Lorentz symmetry is only an approximate one in SVT, the main motivation behind introducing the exact fundamental supersymmetry fades away too. As for the extendedness issue then the theory's goal is to have the nonzero-size feature derived from quantum-mechanical principles rather than mathematically postulated from the beginning - as to be able to answer the "naive" physical questions such as, for example, what is the microscopical structure of the "material" an extended object, be it string or brane or anything else, is "made of". Otherwise such extended object can be regarded only as an approximate, effective description of a real phenomenon.

Logarithmic BEC vacuum theory

In this theory the physical vacuum is conjectured to be the strongly-correlated quantum Bose liquid whose ground-state wavefunction is described by the logarithmic Schrödinger equation. It was shown that the relativistic gravitational interaction arises as the small-amplitude collective excitation mode whereas relativistic elementary particles can be described by the particle-like modes in the limit of low momenta.[15] The essential difference of this theory from others is that in the logarithmic superfluid the maximal velocity of the phonon-like fluctuations is constant in the leading (classical) order. This allows to fully recover the relativity postulates in the "phononic" (linearized) limit.[11]

The proposed theory has many observational consequences. They are based on the fact that at very high velocities the behavior of the particle-like modes becomes distinct from the relativistic one - they can reach the speed of light limit at finite energy.[17] Among other predicted effects is the superluminal propagation and vacuum Cherenkov radiation.[18]

The theory also proposes the mass generation mechanism which may replace or supplement the electroweak Higgs one. It was shown that masses of elementary particles can arise as a result of interaction with the superfluid vacuum, similarly to the gap generation mechanism in superconductors.[11][15] For instance, the photon propagating in the average interstellar vacuum acquires a tiny mass which is estimated to be about 10−35 electronvolt.

See also

Notes

  1. ^ The term "small" refers here to the linearized limit, in practice the values of these momenta may not be small at all.

References

  1. ^ P. A. M. Dirac, Nature 168, 906 (1951).
  2. ^ P. A. M. Dirac, Nature 169, 702 (1952).
  3. ^ K.P. Sinha, C. Sivaram, E.C.G. Sudarshan, Found. Phys. 6, 65 (1976).
  4. ^ K.P. Sinha, C. Sivaram, E.C.G. Sudarshan, Found. Phys. 6, 717 (1976).
  5. ^ K.P. Sinha and E.C.G. Sudarshan, Found. Phys. 8, 823 (1978).
  6. ^ a b G. E. Volovik, The Universe in a helium droplet, Int. Ser. Monogr. Phys. 117 (2003) 1-507.
  7. ^ N.N. Bogoliubov, Izv. Acad. Nauk USSR 11, 77 (1947).
  8. ^ N.N. Bogoliubov, J. Phys. 11, 23 (1947)
  9. ^ V.L. Ginzburg, L.D. Landau, Zh. Eksp. Teor. Fiz. 20, 1064 (1950).
  10. ^ A.D. Sakharov, Sov. Phys. Dokl. 12, 1040 (1968). This paper was reprinted in Gen. Rel. Grav. 32, 365 (2000) and commented in: M. Visser, Mod. Phys. Lett. A 17, 977 (2002).
  11. ^ a b c d e K. G. Zloshchastiev, Spontaneous symmetry breaking and mass generation as built-in phenomena in logarithmic nonlinear quantum theory, Acta Phys. Polon. B 42 (2011) 261-292 ArXiv:0912.4139.
  12. ^ M. Novello, M. Visser, G. Volovik, Artificial Black Holes, World Scientific, River Edge, USA, 2002, p391.
  13. ^ G.E. Volovik, Int. J. Mod. Phys. D15, 1987 (2006) ArXiv: gr-qc/0604062.
  14. ^ L.D. Landau and E.M. Lifshitz, The Classical Theory of Fields, (1951), Pergamon Press, chapter 11.96.
  15. ^ a b c A. V. Avdeenkov and K. G. Zloshchastiev, Quantum Bose liquids with logarithmic nonlinearity: Self-sustainability and emergence of spatial extent, J. Phys. B: At. Mol. Opt. Phys. 44 (2011) 195303. ArXiv:1108.0847.
  16. ^ S.R. Coleman and E.J. Weinberg, Phys. Rev. D7, 1888 (1973).
  17. ^ K. G. Zloshchastiev, Logarithmic nonlinearity in theories of quantum gravity: Origin of time and observational consequences, Grav. Cosmol. 16 (2010) 288-297 ArXiv:0906.4282.
  18. ^ K. G. Zloshchastiev, Vacuum Cherenkov effect in logarithmic nonlinear quantum theory, Phys. Lett. A 375 (2011) 2305-2308 ArXiv:1003.0657.
Central concepts
Black holes
Quantum field theory
in curved spacetime
Proposed theories
others
Toy models
Applications
Others